In the present work, the gravitational discrepancies observed in disk galaxies are explained using a new relativistic theory beyond the standard model of physics. It assumes the existence of bosonic quasi-particles of a scalar field, which reach different relativistic velocities v < v(max) < c depending on her effective mass.
The magnitude of the limit velocity v(max) is derived from a hypothetical non-singularity condition of a quasi-particle at the velocity limit. Remarkably, it turns out that a good fit of the rotation curves is usually achieved by a relativistic velocity distribution of a galaxy-specific quasi-particle, where the maximum rotational velocity at the edge of a disk galaxy is approximately equal to the limit velocity v(max). From the relativistic velocity distribution a galaxy-specific halo with limited mass and finite size can be derived. The way dark matter can be characterized with the new approach suggests that entanglement and quantum correlation of quasi-particles plays a crucial role in the formation of a dark matter halo. To verify the model, 12 representative galaxies were selected from the SPARC data set [astroweb.cwru.edu/SPARC/] and evaluated according to the new relativistic model using the least squares regression method. A comparison of the obtained data with the empirical law of the radial acceleration relation shows high agreement. The SBM theory, which is based on a modification of special relativity theory, is applied here as a first-order approximation for bosonic quasi-particles in the asymptotic flat space of disk galaxies.
3. Results
4. Discussion
5. Conclusion
6. Acknowledgement
7. References
8. Appendix
Abstract
In the present work, the gravitational discrepancies observed in disk galaxies are explained based on a new quantum gravitation theory beyond the Standard Model of physics. It assumes the existence of bosonic quasi-particles of a scalar field with different relativistic velocities v < vmax < c. The extent of the limiting velocity vmax is derived from a hypothetical non-singularity condition of a quasi-particle. Remarkably, it turns out that a modified Maxwell-Jüttner velocity distribution of a galaxy-specific quasi-particle gives a good fit to the observable rotation curves. It is thereby found that the maximum observable rotational velocity at the edge of a disk galaxy approaches the relativistic limiting velocity vmax. The way dark matter can be characterized with the new framework suggests that entanglement and quantum correlation of quasi-particles plays a crucial role in the formation of a dark matter halo. The experimental and theoretical studies provided plausible evidence for the existence of a continuous many-body Floquet state of dark matter in galaxies.
To test the model, 12 representative galaxies were selected from the SPARC data set [astroweb.cwru.edu/SPARC/] and evaluated using the least squares method according to the new model. A comparison of the obtained data with the empirical law the of Radial Acceleration Relation (RAR) shows high agreement.
1. Introduction
An essential feature of A. Einstein’s special theory of relativity [1] is the central statement about the relativity of simultaneity. In the inertial system with time t the proper times τ (1) of two bodies moving towards each other at different speeds v are not synchronously. So, an instantaneous interaction between these two bodies is not possible but runs causally and at most at the speed of light.
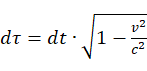 (1)
(1)
On the other hand, quantum mechanics with its interpretation of the non-locality of particles is fundamentally different from Einstein’s view. Consequently, there is what Einstein called a “spooky long-range effect” in the quantum world, which occurs instantaneously, no matter how far apart the partner particles involved are. Nevertheless, the violation of Bell’s Inequality [2] could refute Einstein’s assumption that there could be a theory with hidden parameters that realistically maps quantum mechanical behavior locally [3]. Considering the inconsistencies of ΛCDM Standard Model [4] on the length scale of a galaxy, the influence of quantum gravity seems to have received little attention so far. To date, there is still no bridging theory between quantum mechanics and relativity. The difficulty to understand the physical background of dark matter could be closely related to this. The Schwarzschild de Broglie modification of the special theory of relativity, briefly SBM-model [5], is an attempt to fill this gap with a quasi-particle of ambiguous nature. In a system of quasi-particles with different limiting velocities vmax,i < c, as outlined above, there are more possibilities to establish entanglement between particles. For example, in the same reference system the proper times (2) of different particles can be the same at constant βi, equation (3), i.e., despite different velocities vi and vmax,i they can be synchronous and thus enable simultaneity.
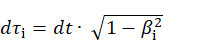 (2)
(2)
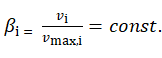 (3)
(3)
As will be shown during the investigation, the βi values of the quasi-stationary Hamiltonian approach a constant value for all quasi-particles(i) in a region of the weak gravity. The idea of particles with different limiting velocities may be their greater potential to generate entanglement. Thus, there could well be a bridge between quantum mechanics and relativity in the form of particles whose physical properties are between the two main pillars of current physics.
The aim of this investigation is to verify such a connection based on experimental data. The paper is structured as follows: In the theoretical part, the physical conditions are described which lead to a limit vmax < c for a specific quasi-particle.
A model presentation is developed, which proposes a Clifford torus as a geometric boundary configuration in the Euclidean 3D part of Minkowski space (Fig. 1). The calibration of the model is based on a concrete estimate belonging to the shape of the Clifford torus compared to the Schwarzschild radius. In the experimental part, the results are presented for 12 galaxies obtained based on the new model with a total of three parameters by least squares method. The results include the distribution of dark matter within a galaxy as well as information on the absolute size and radial density of the dark matter halo. Furthermore, some scaling relations valid in the SBM framework were formulated and compared with the empirical law of radial acceleration relations [6].
2. Theory
There are many theoretical approaches in the literature that share some features with the SBM model presented here [7-9]. This concerns the attempt to describe dark matter within a non-local theory in connection with the properties of special particles. [10–16]. As far as I know, the approach pursued here is the first to assume hypothetical quasi-particles which, when coupled to a gravitational field, exhibit a hierarchical structure of limit velocities that are smaller than the speed of light. Main features of the Schwarzschild de Broglie modification of special relativity (SBM-model for short) were already published [5]. In the meantime, the model has been further developed, moreover it has reached a state that allows an evaluation of the rotation curves of disk galaxies and a comparison with other theories. A summary of the abbreviations and acronyms used in this paper is presented below.
Table 1: Abbreviations:

2.1 SBM-model
Despite great efforts, it has not yet been possible to detect dark matter in the form of unknown elementary particles. This fact [17] points to a physical background which has been largely ignored so far. In this context, the SBM model attempts to take a different approach from the Standard Model. It is based on the idea that dark matter consists of quasi-particles whose modified relativistic properties are determined by an intrinsic gravity. This special form of relativity is determined by the (effective) mass of a quasi- particle. It is assumed to be non-singular bosonic quantum particles of a scalar field, which are not subject to decoherence or thermalization and therefore escape direct measurement. Another assumption concerns the physical character of the particles. It is known that particles with an integer spin can form a Bose-Einstein condensate (BEC) at temperatures around absolute zero. In a BEC, the particles occupy one and the same energy state and create a new quantum mechanical entity due to their indistinguishability [18,19]. In comparison, the SBM model predicts the formation of relativistic condensates along the quasi-stationary Hamiltonian, see Fig. 2a and 3, red solid line. Here, the trajectories of different quasi-particles pass through quasi-stable states of the Hamiltonian. Their formation would not necessarily require temperatures around absolute zero, but rather a certain constant relativistic particle velocity that depends on the effective mass of a quasi-particle. These findings will be discussed in more detail below and in Sections 2.2 and 4.
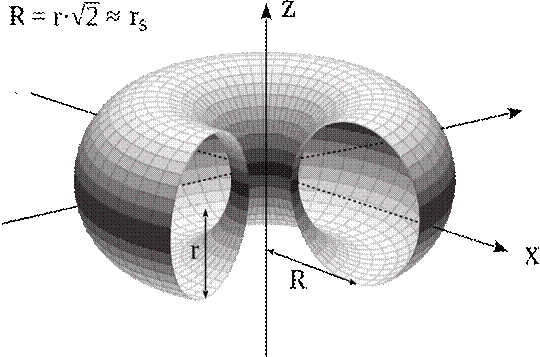
Figure 1: Representation of a quasi-particle
at the speed limit 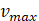 by a stereo graphic
projection of a Clifford Torus into the 3-dimensional Euclidean space. The
ratio of the large (R) to small radius (r) is
by a stereo graphic
projection of a Clifford Torus into the 3-dimensional Euclidean space. The
ratio of the large (R) to small radius (r) is 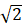 . The
propagation is in the direction of the z-axis.
. The
propagation is in the direction of the z-axis.
The question arises how the limiting velocity of a single quasi-particle of scale mass m can be derived. From the point of view of an observer at rest, the temporal dynamics of a particle comes to a standstill near its relativistic limiting velocity, while at the same time the four-dimensional Minkowski spacetime at the velocity limit is reduced by length contraction to three spatial dimensions of the Euclidean subspace. The state of the quasi-particle at
the velocity limit therefore appears less complex to an observer at rest and is probably best suited for developing concrete ideas about it. To avoid misunderstandings at this point, it should be mentioned that in this study a quasi-particle is regarded as quantum particle with a superposition of many energetically different states. In this sense, a quasi-particle with a certain velocity is only one of many possible energy states.
There are good reasons to choose for the velocity limit a torus whose radius R (Fig. 1) is about as large as the Schwarzschild radius (4). Thus, according to equation (6), a lower limit for the de Broglie wavelength, equation. (5), can be postulated. It allows a minimum of uncertainty and thus should guarantee the conservation of the wave properties of a quasi-particle also at the velocity limit.
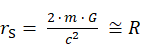 (4)
(4)
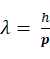 (5)
(5)
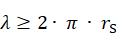 (6)
(6)
Among the four-dimensional flat tori T2 the Clifford Torus
plays a prominent role [20, 21], see Fig. 1. Embedded in the three-sphere 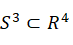 , it represents a minimal
surface with a constant mean curvature. The distances from the center of the unit
sphere to the surface of the torus in
, it represents a minimal
surface with a constant mean curvature. The distances from the center of the unit
sphere to the surface of the torus in 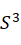 are all equal and are of the
unit length 1. Therefore, the surface of a 4D-Clifford torus would be
advantageous for the formation of a bosonic field quantum, since one and the
same equipotential surface can be occupied. However, this also results in
differences to the SRT. From the point of view of an observer at rest, a
contraction in length must also be followed by a contraction in the transverse
direction of movement to maintain the energy geometrical constraints. In the
Euclidean part of the Minkowski space, however, the contractions are not
uniform but directional, see Fig. 1. A 4D-Clifford torus can be imaged by
stereographic projection into the Euclidean part of the Minkowski space [22,23]
and generates a conformal 3D image, Fig. 1. Of all the flat tori
are all equal and are of the
unit length 1. Therefore, the surface of a 4D-Clifford torus would be
advantageous for the formation of a bosonic field quantum, since one and the
same equipotential surface can be occupied. However, this also results in
differences to the SRT. From the point of view of an observer at rest, a
contraction in length must also be followed by a contraction in the transverse
direction of movement to maintain the energy geometrical constraints. In the
Euclidean part of the Minkowski space, however, the contractions are not
uniform but directional, see Fig. 1. A 4D-Clifford torus can be imaged by
stereographic projection into the Euclidean part of the Minkowski space [22,23]
and generates a conformal 3D image, Fig. 1. Of all the flat tori 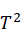 embedded in
embedded in 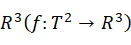 , the Clifford torus is the one
with the lowest Willmore energy W(f) and comes closest
to the elastic bending energy of a minimal surface (2π2) [20]. This
conjecture was originally formulated by Th. Willmore [24,25] and was
mathematically proven in 2012 by Fernando C. Marques and
, the Clifford torus is the one
with the lowest Willmore energy W(f) and comes closest
to the elastic bending energy of a minimal surface (2π2) [20]. This
conjecture was originally formulated by Th. Willmore [24,25] and was
mathematically proven in 2012 by Fernando C. Marques and 
2.2 Speed limit of bosonic quasi-particles
As already shown in section 2.1, for a resting observer a 3D-Clifford torus in the Euclidean part of the Minkowski space R3 is an adequate image of a quasi-particle at the limiting velocity. The geometry of a Clifford Torus at these limit serves here as a basic orientation, see also Fig.1. To calculate the limiting velocity vmax,i, the dynamics of the length contraction of a quasi-particle is being analyzed from the point of view of an observer at rest. Considering the equations (4), (5) and (6) as well as the Lorentz factor (7), a determining equation (8) for the maximum limiting velocity vmax,i of a quasi-particle of mass mi is obtained for the lower limit of λ (6).
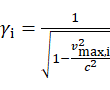 (7)
(7)
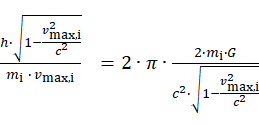 (8)
(8)
The solution for the limit velocity 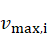 of a quasi-particle of mass
of a quasi-particle of mass 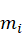 is:
is:
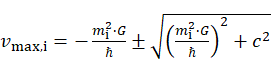 with |vmax,i|
< c (9)
with |vmax,i|
< c (9)
The scale mass mi of a quasi-particle can be an arbitrary quantum. Only solutions with | vmax,i | < c are considered. The energy scaling of the particles is now done in a comparably way as in special relativity (SRT), with the difference that instead of the speed of light the limiting speed vmax,i is considered, see equation (10). Ei is the energy of a free and uniformly moving quasi-particle with velocity v.
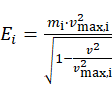 (10)
(10)
The constant velocity trajectories, Fig. 2(a) are remarkable in terms of their energy profile.
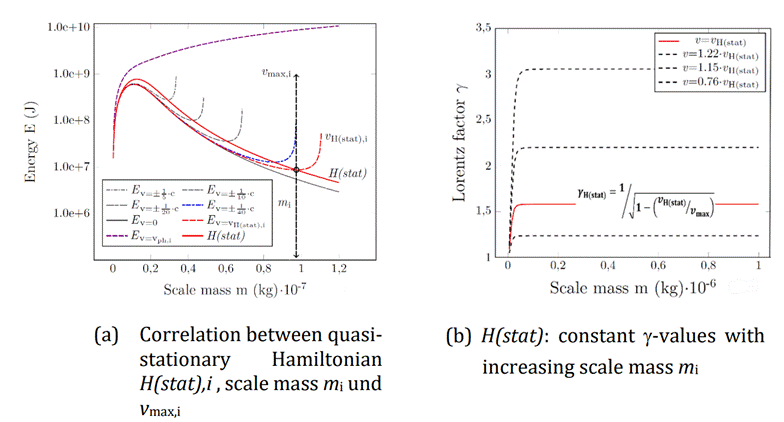
Fig. 2: (a) Scale mass vs. Log. v-trajectories and (b) Scale mass vs. Lorentz factor g
For all quasi-particles in question, the Hamiltonian H(stat),i passes through a minimum required to form a relativistic Bose-Einstein condensate. The formation of a quasi-stationary Hamiltonian is an important feature in the regime of SBM. An analogous behavior is not possible within the framework of Einstein's special theory of relativity (SRT) because of the uniformly valid upper velocity limit c. However, compared to Bose-Einstein condensates, the formation of a relativistic condensate would not require very low temperatures, but a specific relativistic particle velocity. Thus, there would be no restriction to very light particles that can thermalize below about 3 K, such as a hypothetical axion [27,28]. According to the SBM model, a relativistic Bose-Einstein condensate can be formed from quasi-particles with a scale mass m ≥ 1.26∙10-8 kg (effective mass 6.52∙10-9 kg). This will be discussed in more detail in section 4.
The violet curve in fig. 2a marks the phase boundary to the SRT regime, i.e., above this boundary the corresponding particles are energetically more favorable under SRT. According to SBM model, a quasi-particle can be treated as a topological soliton arising from spontaneous symmetry breaking. For each quasi-particle with mass m there is a phase boundary velocity, see appendix 8.1.
Fig. 2(b) shows the variation of the Lorenz factor γH(stat),i
(red) with increasing scale mass according to equation (11). Above a scale mass
of about 2.6e-7 kg, the γH(stat),i values are nearly constant, which may be a
prerequisite for the generation of local entanglement and condensation
formation, as shown at the beginning. This is also true for arbitrary γi values
of different quasi-particles if the velocities vi are composed of a product of
the form a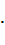 vH(stat),i with a=constant, see Fig.
2b, dashed curves.
vH(stat),i with a=constant, see Fig.
2b, dashed curves.
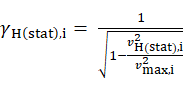 (11)
(11)
Fig. 3 shows the relationship of the quasi-stationary Hamiltonian H(stat), red curves, to an arbitrary elected velocity trajectory vi=vH(stat),i, blue, solid curves, in 3D. P1 and P2 are points on the quasi-stationary Hamiltonian H(stat), which belong to a galaxy-specific quasi-particle of the scale mass mi. The velocity dispersion of the quasi-particle then assumes values between −vmax,i < vi < +vmax,i (black, dashed curve).
A modified Maxwell-Jüttner velocity distribution is used to
calculate particle probabilities in various Newtonian orbits. The choice of
this velocity distribution is motivated by the locally flat space given by the
toroidal (flat) topology of a quasi-particle (see section 2.3). From there, the
space could also be called pseudo-gravitation free. The modified
Maxwell-Jüttner velocity distribution is supposed to apply here. Thus, the
difference to a baryonic particle does not result from their gravitational
embedding, but from the relativistic distribution.
For the gravitational embedding of a quasi-particle at a certain distance from
the center of a galaxy, the minima in Figs. 2a and 3 for the Hamiltonian
H(stat) at constant velocity are of particular interest. They indicate a
quasi-stable state in a circular orbit. Therefore, it is useful to know the
analytical solution for the minima of the Hamiltonian H(stat).
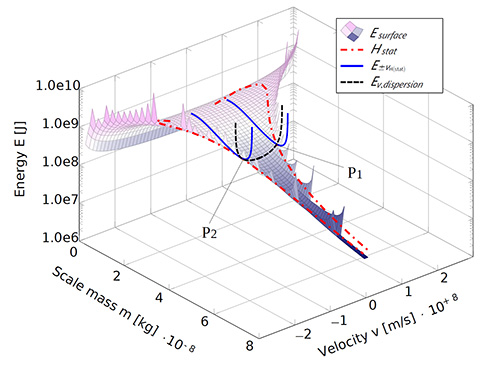
Figure 3: 3D representation of the velocity dispersion of a quasi-particle of mass mi (black, dashed)
A solution (12) for H(stat),i can be found via a variation calculus which depends only on vmax,i. See also equation (14), Fig. 2(a) and Fig. 3, red solid curve.
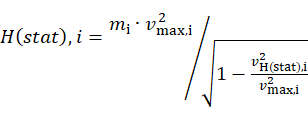
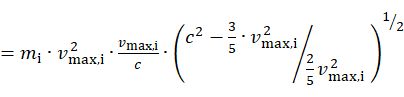 (12)
(12)
In section 2.3 equation (12) is used to derive an expression for the relativistic velocity distribution of a quasi-particle. From (12) a direct relationship can be established between the limit velocity vmax,i and the associated velocity vH(stat),i , equation (13):
- Quote paper
- Siegfried Gantert (Author), 2020, A Non-Standard Relativistic Approach for Simulating Disk Galaxies, Munich, GRIN Verlag, https://www.hausarbeiten.de/document/947925



